How To Find The Area Of A Trapezoid With Fractions

Area of a trapezoid

This lesson volition show you how nosotros notice the area of a trapezoid using two different methods.

Cutting up a trapezoid and rearranging the pieces to make a rectangle and a triangle.

Using the formula for finding the expanse of trapezoids.

The first method will aid you lot meet why the formula for finding the area of trapezoids work.

Permit us get started!

Cut the trapezoid below in three pieces and make a rectangle and a triangle with the pieces.

The figure on the left shows the trapezoid that you need to cut and the figure on the right shows the rectangle and i triangle.

Area of a trapezoid

Area of trapezoid

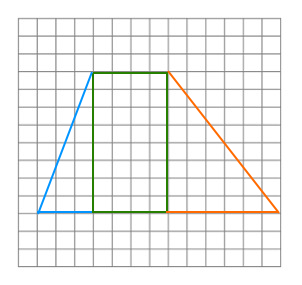
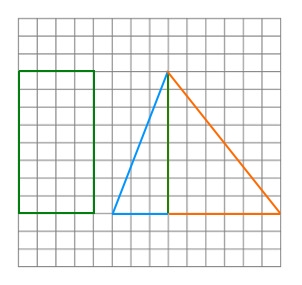
Then, nosotros need to make the following four important observations.

ane.

Rectangle

Base of operations = 4
Tiptop = 8

ii.

Trapezoid

Length of lesser base = 13
Length of top base = 4
Height = eight

iii.

Newly formed triangle (made with blue and orange lines)

Length of base = 9 = 13 - four = length of bottom base of trapezoid - iv
Tiptop = 8

iv.

Area of trapezoid = area of rectangle + area of newly formed triangle.

Now our strategy volition be to compute the area of the rectangle and the area of the newly formed triangle and see if we can brand the formula for finding the area of trapezoid magically appear.

Expanse of rectangle = base × height = iv × eight

Area of triangle = ( base × height ) / 2

Expanse of triangle = [(13 - 4) × 8 ] / 2 = [13 × 8 + - 4 × eight] / two

Area of triangle = (13 × 8) / 2 + (- 4 × eight) / 2

Expanse of trapezoid = iv × eight + (13 × viii) / 2 + (- four × viii) / ii

Area of trapezoid = 8 × (iv + thirteen / two + - four / 2)

Surface area of trapezoid = viii × (4 - 4 / 2 + thirteen / ii)

Area of trapezoid = 8 × (viii / 2 - 4 / 2 + xiii / ii)

Area of trapezoid = 8 × (4 / 2 + 13 / 2)

Area of trapezoid = (4 / ii + 13 / ii) × 8

Area of trapezoid = 1 / 2 × (4 + 13 ) × 8

Permit b₁ = 4 let b₂ = 13, and permit h = 8

Then, the formula to get the surface area of trapezoid is equal to one / 2 × (b₁ + b_ii ) × h

Formula for finding the area of a trapezoid

In general, if b_one and b₂ are the bases of a trapezoid and h the elevation of the trapezoid, then we can use the formula below.

Area of a trapezoid formula

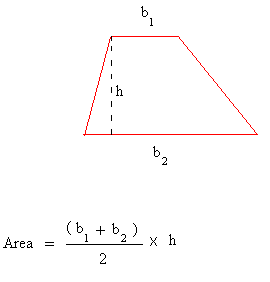
Examples showing how to find the area of a trapezoid using the formula

Example #one:

If b₁ = vii cm, b_two = 21 cm, and h = 2 cm, find A

Expanse = 1 / 2 × (b_i + b₂ ) × h = i / 2 × (7 + 21) × 2 = one / two × (28) × 2

Area = one / 2 × 56 = 28 cm²

Example #2:

If b₁ = 15 cm, b₂ = 25 cm, and h = 10 cm, find A

Area = one / 2 × (b₁ + b₂ ) × h = 1 / two × (xv + 25) × 10 = i / two × (twoscore) × 10

Area = one / two × 400 = 200 cm²

Example #iii:

If b_one = 9 cm, b_two = 15 cm, and h = two cm, find A

Area = i / two × (b_i + b_two ) × h = ane / 2 × (9 + 15) × two = 1 / two × (24) × 2

Area = i / 2 × 48 = 24 cm²

Area of a trapezoid quiz to notice out if you really empathise this lesson.

Purchase a comprehensive geometric formulas ebook. All geometric formulas are explained with well selected discussion problems so you can master geometry.

Geometry ebook