How To Find The Average Speed Of An Object

Average Speed Formula

Average speed is the mean value of the speed of a body over a period of time. The formula for average speed is needed since the speed of a moving body is not abiding and varies across a menstruum of fourth dimension. Fifty-fifty with varying speed, the values of full time and the full distance covered can be used, and with the help of the formula for average speed, we can find a single value to correspond the entire motion.

What Is the Average Speed Formula?

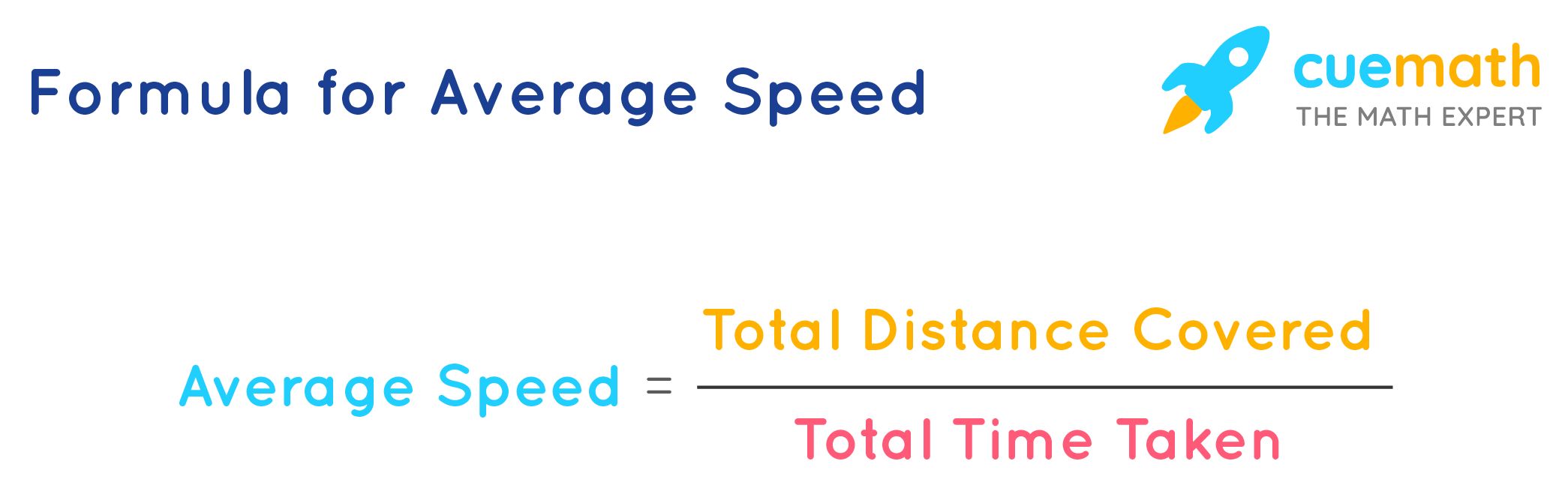
The average speed of a body is equal to the total distance covered, divided by the total time taken. The formula for average speed is given equally:

Average Speed Formula:

Average Speed = Total altitude covered ÷ Total time taken

The average speed formula

Average Speed Formula Special Cases

Instance 1: For a body or object traveling with a speed of \(s_1 \) for time \( t_1 \), and the speed of \(s_2 \) for time \( t_2 \), the formula for average speed is given in the below expression. The production of \(s_1 \times t_1 \), and \(s_2 \times t_2 \) gives the distances covered in time intervals \(t_1 \), and \(t_2 \) respectively.

Average Speed Formula \(= \frac{s_1 \times t_1 + s_2 \times t_2}{t_1 + t_2}\)

Case 2: Similarly, when 'due north' dissimilar speeds, \(s_{i}, s_{2}, s_{three},... s_{due north}\), are given for 'north' respective individual fourth dimension intervals, \(t_{ane}, t_{two}, t_{3},... t_{northward}\) respectively, the average speed formula is given equally:

Average Speed Formula \(= \frac{s_1 t_1 + s_2 t_2 + ... + s_n t_n}{t_1 + t_2 +...+ t_n}\)

Case 3: Average speed when different distances, \(d_{i}, d_{2}, d_{iii},... d_{north}\), are covered for unlike intervals of time, \(t_{1}, t_{2}, t_{3},... t_{north}\) repectively is given as:

Average Speed Formula \(S_{avg}\) \(= \frac{d_1 + d_2 + d_3 +...+ d_n} {t_1 + t_2 + t_3 +....+ t_n}\)

Case 4: Average speed when different speeds, \(s_{one}, s_{2}, s_{iii},... s_{north}\), are given for different distances, \(d_{i}, d_{2}, d_{iii},... d_{n}\) respectively is given as:

Boilerplate Speed Formula \(S_{avg}\) \(= \frac{d_1 + d_2 + d_3 +...+ d_n} {\dfrac{d_1}{s_1} + \dfrac{d_2}{s_2} + \dfrac{d_3}{s_3} +....+ \dfrac{d_n}{s_n}}\)

Instance five: Boilerplate speed formula when ii or more than speeds are given (\(s_{1}, s_{ii}, s_{3},... s_{n}\)) such that those speeds were traveled for same amount of fourth dimension (\(t_{1} = t_{2} = t_{iii} =... t_{due north} = t)\) is given equally:

Average Speed Formula, \(S_{avg}\) \(= \frac{s_{i} t + s_{2} t +...+ s_{n} t} {t\times n} = \frac{s_{1} + s_{two} +...+ s_{northward}} {n} \)

Example 6:Average speed when different speeds given (\(s_{1}, s_{2}, s_{3},... s_{n})\) for aforementioned distance (\(d_{1} = d_{2} = d_{3} =... d_{northward} = d)\) is given as:

Average Speed Formula \(S_{avg}\) \(= \frac{ n \times d} { d \times \left[ \dfrac{ane}{s_1} + \dfrac{1}{s_2} + \dfrac{1}{s_3} +....+ \dfrac{1}{s_n}\right]} = \frac{ due north} {\left[ \dfrac{i}{s_1} + \dfrac{1}{s_2} + \dfrac{1}{s_3} +....+ \dfrac{ane}{s_n}\correct]}\)

Desire to find complex math solutions within seconds?

Use our free online computer to solve challenging questions. With Cuemath, find solutions in simple and easy steps.

Book a Gratis Trial Class

Examples on Average Speed Formula

Permit us take a await at a few examples to better sympathise the formula for average speed.

Example 1: Using the average speed formula, find the average speed of Sam, who covers the first 200 kilometers in 4 hours and the next 160 kilometers in another 4 hours.

Solution:

To observe the boilerplate speed nosotros need the total distance and the total time.
Total distance covered by Sam = 200Km + 160 km = 360 km
Full fourth dimension taken by Sam = 4 hour + four hr = eight hour
Average Speed = Full altitude covered ÷ Total time taken
Average Speed = 360 ÷ eight = 45km/hr

Answer: Average speed of Sam is 45 km/hr.

Case 2:A train is moving with a speed of 80 miles per hour for the first four hours and 110 miles per hour for the next three hours. Find the average speed of the train with the help of the average speed formula.

Solution:

It is given that the train is moving at a speed of 80 miles per hr for the offset 4 hours.
Here \(S_1 \) = eighty and \(T_1 \) = four.
And the train is moving at a speed of 110 miles per hr for the next iii hours.
Hence \(S_2 \) = 110 and \(T_2\) = iii.
Boilerplate Speed Formula = \(\frac{S_1 \times T_1 + S_2 \times T_2}{T_1 + T_2}\)
Average Speed = (80 × four + 110 × 3) ÷ (4 + iii)
= (650) ÷ (7) = 92.86 miles/hr

Answer: The average speed of the railroad train is 92.86 miles/60 minutes.

Example iii:A car travels at a speed of 45 km/hr for 5 hours and then decides to slow down to 40 km/hour for the side by side 2 hours. Calculate the average speed using the average speed formula.

Solution:

Distance I = 45 × v = 225 miles
Distance II = 40 × 2 = 80 miles
Total altitude = Altitude 1 + Distance 2
D = 225 + lxxx = 305 miles
Using average speed formula = Total distance traveled ÷ Total time taken
Average Speed = 305 ÷ vii = 43.57 m/s.

Answer:Average speed of a car is 43.57 m/s.

FAQs on Average Speed Formula

How To Calculate Distance Using Average Speed Formula?

The general formula for average speed is given as [Average Speed = Distance Traveled ÷ Full Fourth dimension Taken]
To calculate the distance, the boilerplate speed formula can be molded as [Distance = Average Speed × Fourth dimension].

How To Calculate Time Using Average Speed Formula?

The general average speed formula is given as [Average Speed = Distance ÷ Time]
To calculate the time, the average speed formula will be molded as [Time = Distance Travelled ÷ Average Speed].

How To Use the Formula for Average Speed?

To understand how to use the formula for boilerplate speed let u.s. consider an example.
Example: A runner completes a 100m lap in xl sec. Afterwards the finish of the first lap, he reached back to the starting point. Calculate the boilerplate speed of the runner.
Solution: Full distance covered by the runner = 100 meters
Total time = 40 sec
Then, applying the general formula for the average speed
we take,
Average Speed = Altitude ÷ Fourth dimension
Average Speed = 100 ÷ 40 = two.5m/southward.
The average speed of the runner is two.5m/s.

What will Exist the General Average Speed Formula for an Object?

The general speed average formula for an object is given as [Average Speed = Full Altitude Traveled ÷ Total Time Taken]. SI unit of boilerplate speed is chiliad/south.